Clarity7 Electrical Performance
Technical analysis and measurements
In order to establish a baseline for comparison, we simulated and measured a 4.86 meter (16 feet) section of 11 AWG (American Wire Gauge) ZIP cord and the same length Clarity7 speaker cable. The simulation models were generated using a finite-element field solver and the simulations were done using both SPICE3 and HSPICE. These tools take into account all of the relevant effects described in Maxwell's electromagnetic wave equations, rather than the inaccurate approximations of simple circuit-solvers. The models are distributed lumped lossy models with coupling between all conductors. The physical cross-sections of the 11 AWG ZIP and the Clarity7 that were used to generate the models are shown below:
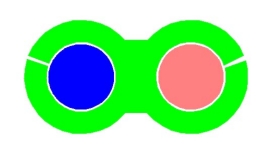
11 AWG ZIP cord (PVC insulated)
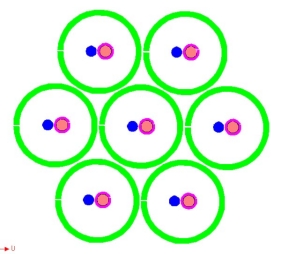
Clarity7 11 AWG equivalent (Teflon insulated)
To compare the performance of Clarity7 to 11 AWG ZIP-cord, we need to examine frequency response, phase response, transient response and understand the resonant characteristics of both. All of these are important characteristics for audio.
Frequency Response Simulations
Frequency response plots were generated from simulations for the ZIP and Clarity7 when driven from an ideal solid-state amp with .2 ohm output impedance and an infinite bandwidth. The plot of Figure 1 shows the response of both cables:
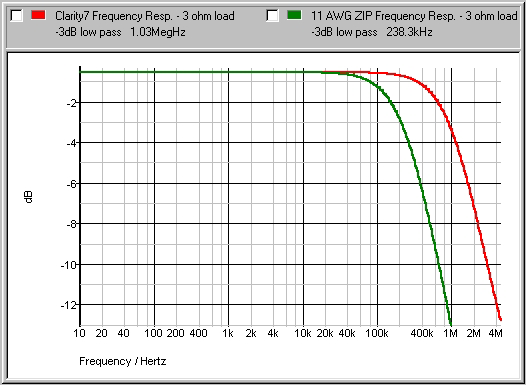
Figure 1
Figure 1 shows that the frequency response of the Clarity7 is more than 4X that of the 11 AWG ZIP-cord. The -1db point of the ZIP is about 70KHz, whereas the Clarity7 -1dB point is at 300KHz. From this result you can see that the 11 AWG barely stays linear through the audio range, falling off dramatically after 20KHz. Remember, this is the attenuation of the cable alone, with no other contributors. Most amplifiers do not have infinite bandwidth and speakers have their own high-frequency response roll-off, so these will only add to the attenuation that is caused by the cable. Therefore, to minimize overall high-frequency attenuation, the cable should be as flat as possible through the audio range.
Time-Domain Measurements
So what kind of effect will this have on music waveforms? To illustrate this, we made actual measurements of a system consisting of a CODA 10.5 amplifier driving each of the 16 foot cables to a KEF 104/2 loudspeaker load. The waveform is a 9 KHz square-wave at relatively low-level (to avoid tweeter damage through resonance). The waveform measurements of Figure 2 were taken at the KEF loudspeaker binding posts:
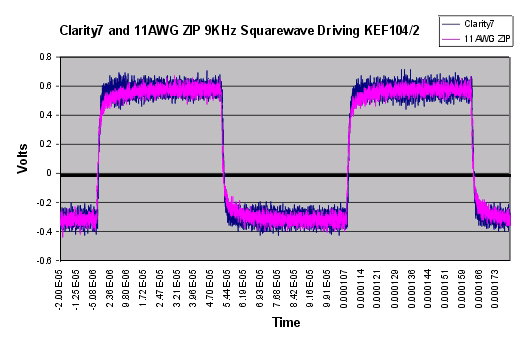
Figure 2
The superimposed waveforms of Figure 2 show clearly that the leading edges of the squarewave are being rounded with the 11 AWG ZIP-cord, but the fast leading-edge is preserved in the Clarity7 waveform. There is also high-frequency noise riding on the waveforms because these signals were at relatively low-level (.8V peak-to-peak). Notice that the high-frequency noise is attenuated by the 11 AWG ZIP as compared to the Clarity7. These effects are all evidence of high- frequency roll-off in the 11 AWG ZIP-cord. To more clearly see the difference, the waveforms above were mathematically filtered to remove the high-frequency "fuzz" to create the plot of Figure 3:
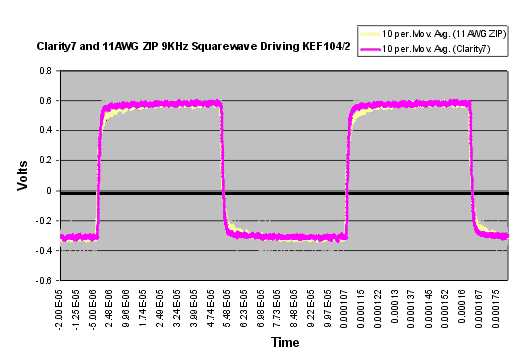
Figure 3
The rounding of the leading edges in the 11 AWG ZIP-cord waveform is clearly visible in Figure 3. The Clarity7 waveshape is virtually identical to the pulse generator output.
Phase Response Simulations
Next, we compare the phase response of the Clarity7 to the 11 AWG ZIP-cord. The graph of Figure 4 performs a simulation of the difference in phase from the input to the output of each of the two cables. This is the phase error:
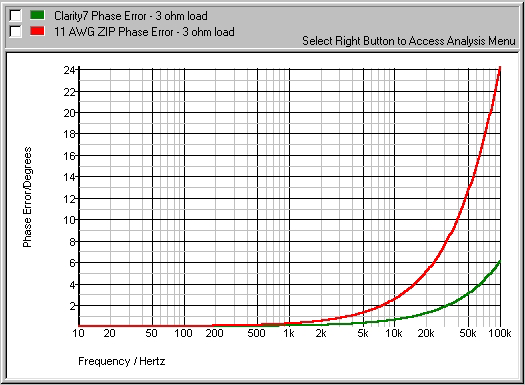
Figure 4
The plot shows that the phase error introduced by the 11 AWG ZIP-cord is more than 5 degrees at 20 KHz. The Clarity7 introduces only 1.3 degrees of phase error at 20 KHz in this case. This really should be the worst-case in any system unless the speaker impedance is lower than 3 ohms. The KEF 104/2 impedance is relatively flat at 3 ohms.
Time-Domain Measurements
To illustrate the effect of phase shift, we made some actual measurements on a system comprised of a CODA 10.5 amplifier driving both cables to a KEF 104/2 loudspeaker load. This time, we use a real music waveform burst: the first few milliseconds of track 6 on the Spiro Gyra "Got the Magic" album. This music burst contains musical transients containing 8 KHz and 16 KHz components. We measured the music burst at the amplifier input (unbalanced) and at the speaker with each cable (Clarity7 and 11 AWG ZIP) connecting them. The following plot shows the waveform of the burst. The AMPIN signal was scaled-up to be the same voltage as the speaker voltage. 15K data points were captured for each waveform of Figure 5 using a Digital Sampling Oscilloscope (DSO) with 500MHz bandwidth (instrument and probes):
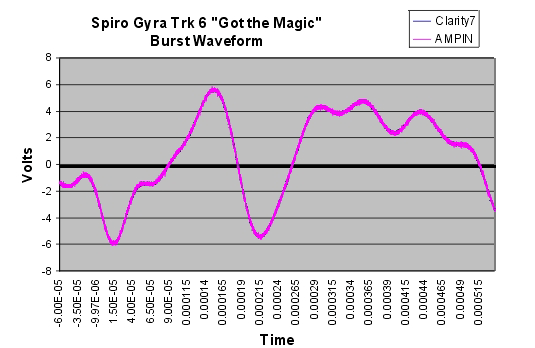
Figure 5
The waveform overlay of Figure 5 shows that the Clarity7 output tracks the amplifier input closely. To easily illustrate differences between the signal at the amp input and the signal at the speaker terminals, we subtract the two signals mathematically in the following plots creating an "error voltage". First the 11 AWG ZIP plot in Figure 6:
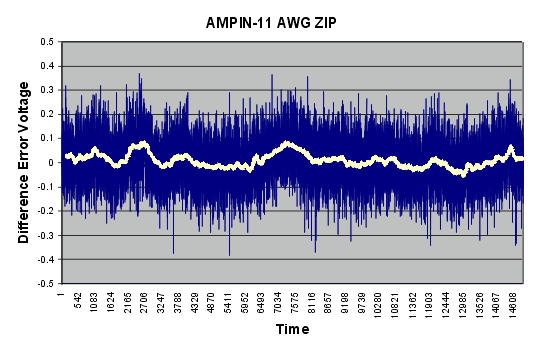
Figure 6
The plot shows that the 11 AWG ZIP is not tracking the input waveform very well, particularly at the high-amplitude transitions in Figure 5. Ideally, the yellow line should be flat. Figure 7 is the same error voltage plot for the Clarity7:
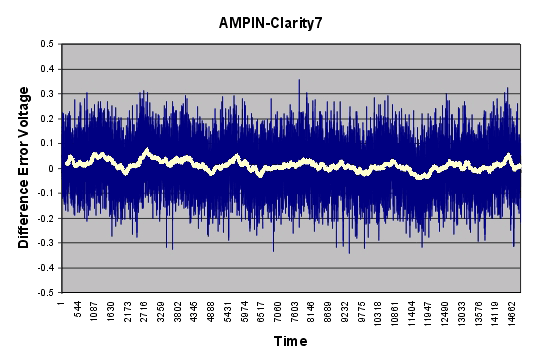
Figure 7
The plot of Figure 7 shows a much flatter difference voltage, indicating that the Clarity7 is not introducting as much phase error as the 11 AWG ZIP-cord. There is likely some quantization error in the calculations, so some of the small peaks are likely a result of that. This means that the samples that were taken for each cable did not occur at exactly the same points in time, so some errors will be present, particularly where the waveform is changing at a high rate.
Transient Response Simulation
Good transient response is critical for accurate music reproduction. Transient response is very difficult to measure with anything other than a square wave, which is shown above. However, using simulation models, it is possible to build systems that would be difficult to implement in real life. These simulations can apply virtually any waveform and frequency to the cable to better understand it's characteristics. In the following simulations, we apply a very fast (1 µsec risetime) step to both the 11 AWG ZIP-cord and the Clarity7 cable. Figure 8 shows the time-domain response of both cables:

Figure 8
It is obvious from Figure 8 that the 11AWG ZIP-cord cannot pass a step voltage this fast. The Clarity7, however reproduces the edge-rate well, with only a small delay. Now, lets look at the spectrum of these waveforms to see how the cables behave in the frequency domain. This is done mathematically by performing a discrete Fast-Fourier Transform (FFT) on the waveform data above. This creates a frequency plot of the spectral energy contained in the voltage step. Figure 9 is the spectral plot of the above voltage step waveforms:
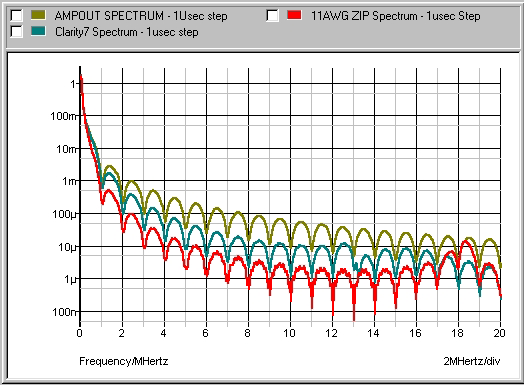
Figure 9
In Figure 9 the higher-order harmonics of the step are visible as humps. The spectrum of the Clarity7 tracks well under 1 MHz and has the same general shape as the amplifier output spectrum above 1 MHz. The 11AWG ZIP, however, diverges from the amp-in spectrum well under 1 MHz and has a "saddle" pattern at higher frequencies that deviates from the shape of the amp-in spectrum significantly. This indicates some type of resonance is occurring. Now, lets zoom-in and take a closer look at what happens below 1 MHz in Figure 10:
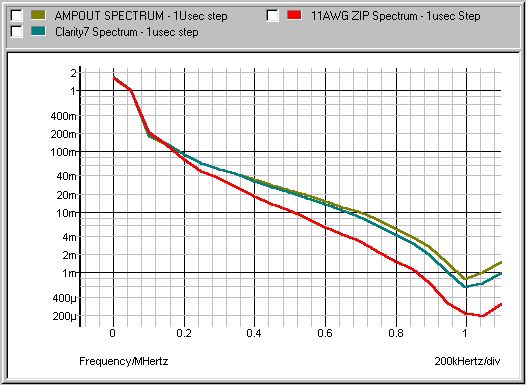
Figure 10
Figure 10 shows that the Clarity7 accurately reproduces the waveform components to at least 1 MHz, whereas the 11 AWG ZIP is having trouble at less than 100KHz. Note that the voltage scale is a log-scale in this case.
Resonance Simulations
Resonance is a phenomena that is typically overlooked in audio cable design. It is always a serious consideration in digital link design, however. All physical systems that are not too lossy exhibit resonance, including cables. See the Audio FAQ for more general information on this. Resonance in audio cables is a function of their length and distributed impedance. We have determined empirically that resonance is audible, not as a primary effect, but that it becomes audible through other secondary effects. Because resonance can cause relatively high-amplitude very high-frequency signals that sustain themselves on the cable, this can apply unexpected voltages and currents to the amplifier output drivers. These voltages can cause the apparent impedance of the cable and speaker to vary beyond what the drivers can handle and still remain linear. Long before non-linearity occurs, the driver circuit's crossover distortion may also increase enough to be audible as well (not an problem in single-ended designs). To explore resonance effects in the 11 AWG ZIP-cord and the Clarity7 speaker cable, simulations were performed to generate frequency response graphs, sweeping the frequency high enough to observe the resonances. Figure 11 is a frequency response plot of the 11 AWG ZIP-cord being driven by an ideal .2 ohm driver into a purely resistive 3 ohm load:
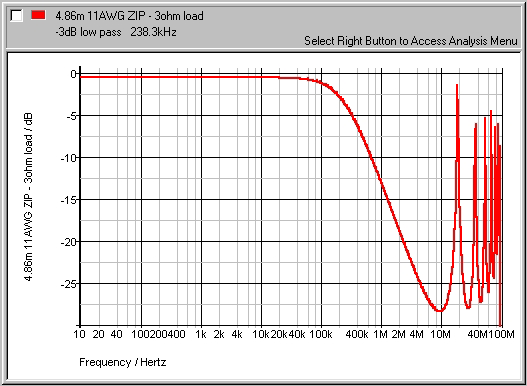
Figure 11
In Figure 11 the fundamental resonance of the 4.86 meter ZIP-cord is at 18 MHz and its harmonics are all visible as well. The fundamental peak is quite high, therefore a resonance might be sustained. Figure 12 shows the same plot for the Clarity7 speaker cable with Anti-Resonance termination:
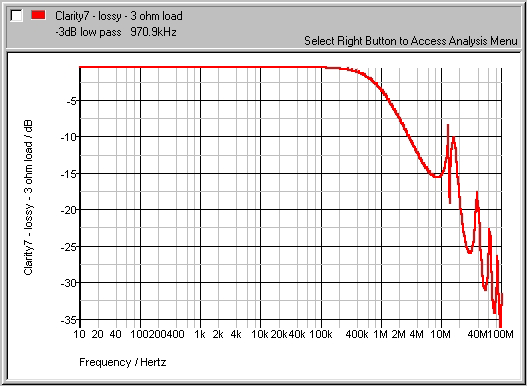
Figure 12
In Figure 12 the fundamental resonant peak is reduced by at least 8 dB and the harmonics are 17-32 dB down. This is how the Clarity7 achieves its "jet-black" background and crisply focused three-dimensional image.