Are cable resonances real and can they be audible?
All physical systems that are not too lossy exhibit resonance. Designing a high-bandwidth cable means that it is a low-loss system by design and therefore prone to resonating. Resonance can be excited by many phenomena. If you pluck a guitar string or strike a bell, they will ring at their natural resonant frequency and then decay over time due to losses in the systems. If you strike a bell with a hammer or only your finger, it will resonate in both cases, just not as high an amplitude with the finger strike. Cables likewise will ring when a transient voltage is applied, their resonant frequency being a function of their length. When resonance occurs in cables, a standing-wave of voltage oscillates the length of the cable causing voltage peaks and valleys that change over time, much like the bowing of a stretched rubber band that is plucked in the middle. If one examines the effect at one end of the cable, there will be a voltage oscillation that rides on top of whatever waveform is otherwise occurring on the cable, music for instance.
Resonance occurs in both interconnects and speakers cables, however, because interconnects are generally much shorter, the resonant frequencies are extremely high and these resonances generally tend to damp-out quickly. The loads at the end of interconnects are usually very high impedance and therefore the drivers are somewhat insensitive to additional load. Speaker cables on the other hand can be very long, lowering the resonant frequency and because the drivers can be load sensitive, this can cause problems. If a high-amplitude resonance occurs on a speaker cable, the driver may have unexpected voltages appear at it's output that make the cable and loudspeaker combination appear to be very low impedance. If the amplifier is not designed to drive very low-impedance loads, say 1-2 ohms, it may saturate, causing distortion. Even if it does not saturate, the circuitry that eliminates crossover-distortion in most amplifiers may not work optimally at high loads. In fact, the crossover distortion in most amplifiers increases with load.
To get a better feel for what resonance is in cables, some SPICE simulations and some measurements were performed. Using a 4.86 meter 11 AWG ZIP cord, a fast (35nsec) voltage step was applied with no load on the cable (end not connected). The following plot shows the SPICE simulation of the waveform at the driver end and the open end of the cable. The 11AWG ZIP cable model described previously was used for the simulation. The driver impedance of the pulse generator in this case was 7.7 ohms:
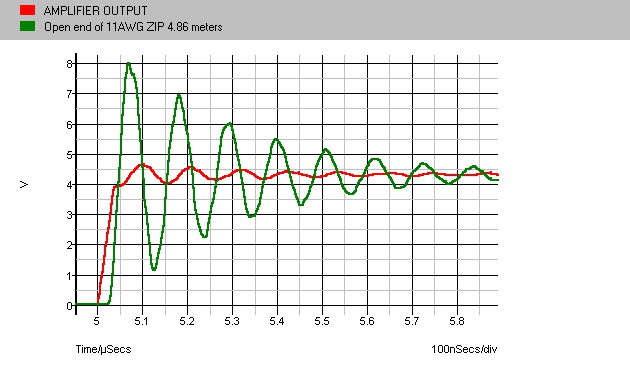
The plot shows that the ringing even on ZIP cord can be quite large and a single event can take up to 1 microsecond to damp-out. The input step was 4.3 volts and the ringing excursions were up to 8 volts. Also notice in the red waveform that the resonance is affecting the driver as well.
Next a measurement of the same situation was taken on the bench with a Digital Sampling Oscilloscope (DSO) to insure that the SPICE model was accurate:
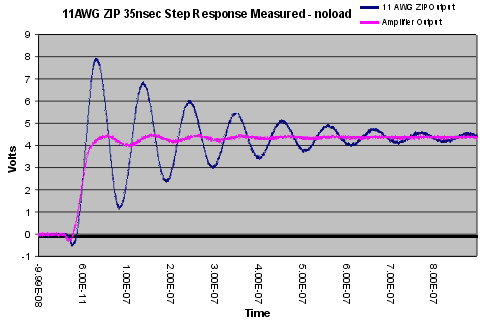
Examination of the simulation and the measured shows that they correlate very closely indeed. This indicates that the simulation model is very close to reality. In both cases , the ringing frequency was the same, at around 9 MHz.
If we do a frequency sweep of the cable with no load, we should be able to see the resonances show up and we would expect the primary one to be located around 9 MHz. The following plot is a simulation frequency sweep (also known as a spectral plot) of the 4.86m 11AWG ZIP cable with no load:
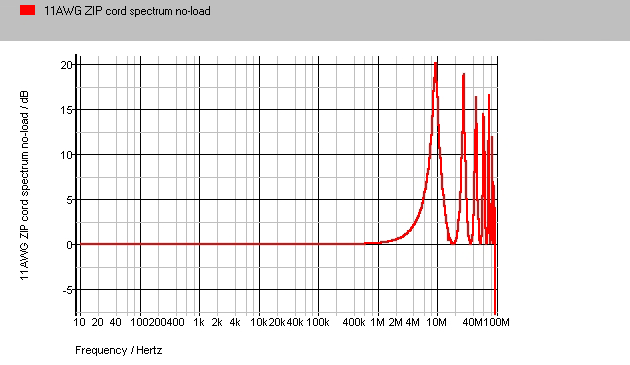
Examination of the plot above shows that the fundamental resonant frequency is located at ~9 MHz as expected. This resonance also shows a 20dB amplification at resonance. This is 100 times the input waveform. Now lets zoom-in and look at the harmonics of this resonance to see if they are even or odd-order:
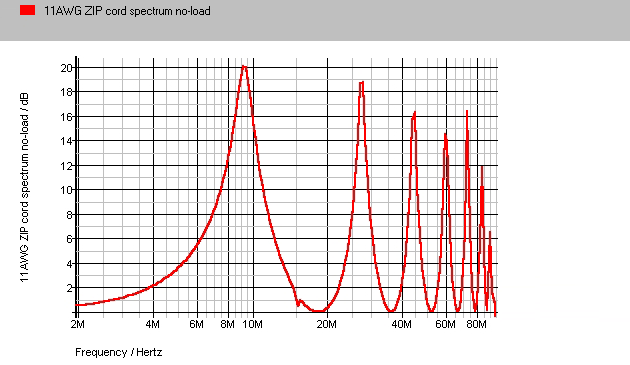
The fundamental resonance is actually at 9.2 MHz. The first harmonic is odd-order and is located at 27.1MHz. The third harmonic peak is at 43.9MHz, which is ~5 times the first harmonic. The fourth harmonic peak is at 59MHz, which appears to be ~6 times the first harmonic. The other peaks are at 72.3MHz, 83MHz and 89.4MHz. So now the interesting question is: what happens to these resonant peaks once a loudspeaker is connected to the end. The following plot is a simulated spectral response of the 4.86m 11 AWG ZIP with a 3 ohm purely resistive load. In this case, the driver has been replaced with a typical amplifier impedance of .2 ohms to simulate a real amplifier/speaker environment:
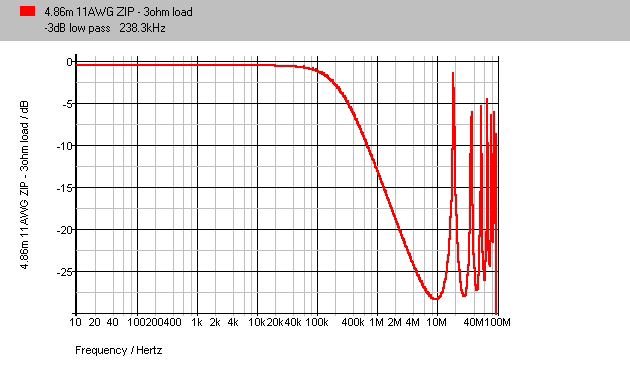
The plot above shows the obvious high-frequency roll-off of the ZIP cable (-3dB at 238KHz), and that the fundamental harmonic has been shifted to 18MHz, exactly twice the frequency of the open-circuit cable. The fundamental harmonic is still high-amplitude, reaching almost -1dB. This means that if the cable were to be stimulated sufficiently, it could ring with the 18MHz resonance at low amplitude, but it would damp out quickly. Higher-order harmonics are now located at 36, 53, 66, 77.7, 87.3 and 93.3MHz. These are mostly -5dB to -8dB down so they are not of particular concern. The resonant frequency is primarily a function of the length of the cable.
Empirical Audio Anti-Resonance Termination
Empirical Audio has taken steps to deal with resonance in our cables. We put anti-resonant terminations in our cables to reduce this effect. To understand the effect of the anti-resonant termination, we first examine a cable without the termination. The following is a spectral plot of our Clarity7 speaker cable driving a 3 ohm load with the anti- resonant termination removed:
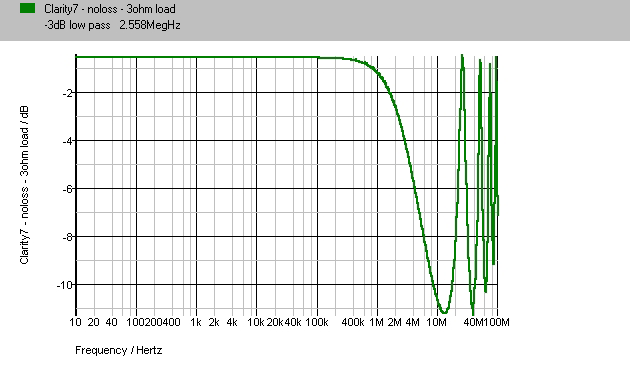
The graph shows the fundamental resonance is similar to the 11AWG ZIP, but the frequency is much higher, at 25MHz. The roll-off is also much higher in frequency than the 11AWG ZIP. The -3dB frequency is at 2.5MHz. Also, the slope of the roll-off is much steeper than the 11AWG ZIP cable. The next plot is the same Clarity7 cable driving the 3 ohm load, but with the anti-resonant termination applied:

The plot above shows that the fundamental resonance is now almost 10dB down. This is 1/10th amplitude. This was accomplished without affecting the audio frequencies. The -3db point for the cable is now at ~1MHz and the roll-off slope is less steep. Empirical Audio cables have very high-bandwidth in order that the anti-resonant terminations can be added. This insures that resonances are virtually eliminated without affecting the audio frequency or phase response.
Real-world speaker load
Next, we look at a real-world loudspeaker load and it's effect on the resonance of the 11AGW ZIP cord speaker cable. The speaker of choice is the Proac Response 1SC, which has a very complex high-order crossover. A SPICE model has been generated for this speaker. First, we plot the impedance of the Proac 1SC speaker model:
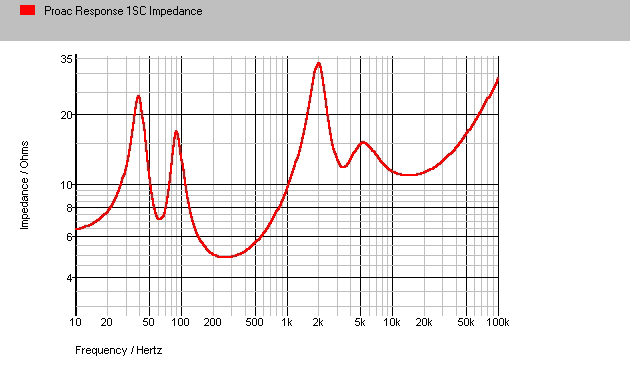
The SPICE model is virtually identical to the impedance curve measured by Stereophile in their March 1998 review. Notable features of this impedance are low dips at 60 and 250Hz and a relatively high impedance for the tweeter. Next we will plot the response of the 11AWG ZIP cord when driving the Proac Response 1SC speaker model:
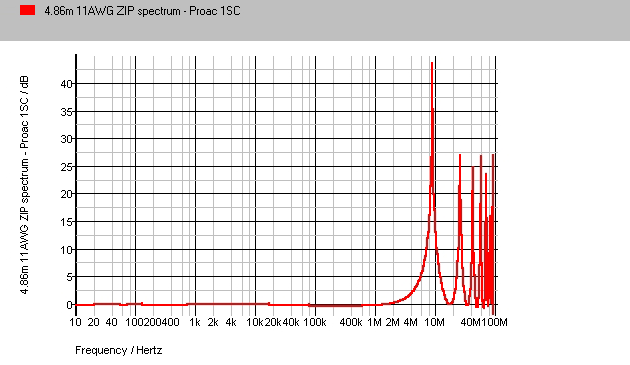
The spectral plot above shows that with a Proac Response 1SC speaker load, the resonance moved back to 9MHz and the resonant peak has doubled in size. 48 dB is 63,000 times magnitude, so this may be a serious resonance problem. The learning to take away from this result is that the resonance is both a function of the driver impedance and the load impedance and that some loads may actually increase resonant behavior significantly.
Conclusions
Resonance is a very real behavior in most speaker cables. The nature of the speaker load will vary both the amplitude and frequency of the resonance. The fundamental resonant frequency is primarily a function of the cable length, but different loads can move this frequency. Resonances can become a problem if high-bandwidth amplifiers are driving high-impedance speaker loads.
Empirical Audio takes resonance seriously, so we design anti-resonant terminations into our cables to eliminate the problem. We believe that the audible "Haze" or "Veils" that often overlay music in high-end systems is in part a result of cable resonance.